Stratified Sampling
Stratified sampling is a probability sampling method and a form of random sampling in which the population is divided into two or more groups (strata) according to one or more common attributes. These attributes can be sex, age, income, level of education etc. according to aims and objectives of the study.
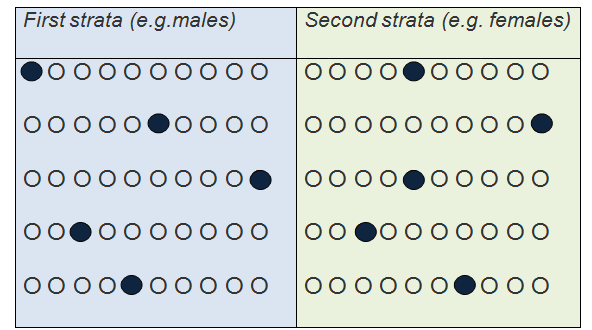
Stratified random sampling intends to guarantee that the sample represents specific sub-groups or strata. Accordingly, application of stratified sampling method involves dividing population into different subgroups (strata) and selecting subjects from each strata in a proportionate manner. The figure below illustrates simplistic example where sample group of 10 respondents are selected by dividing population into male and female strata in order to achieve equal representation of both genders in the sample group.
Stratified sampling can be divided into the following two groups: proportionate and disproportionate. Application of proportionate stratified random sampling technique involves determining sample size in each stratum in a proportionate manner to the entire population. For example, if the entire population for a research is 5000 people, in proportionate stratified random sampling the group can be divided into five strata with 1000 people in each stratum.
In disproportionate stratified random sampling, on the contrary, numbers of subjects recruited from each stratum does not have to be proportionate to the total size of the population. If disproportionate stratified random sampling is applied in a research with 5000 people, the population can be divided into five strata with following unequal numbers of population in each stratum: 1000, 1500, 1200, 800 and 500.
Accordingly, the application of proportionate stratified random sampling generates more accurate primary data compared to disproportionate sampling.
Application of Stratified Sampling: an Example
Suppose, your dissertation aims to explore leadership styles exercised by medium-level managers at Bayerische Motoren Werke Aktiengesellschaft (BMW AG). You have selected semi-structured in-depth interviews with managers as the most appropriate primary data collection method to achieve the research objectives.
Application of stratified random sampling contains the following three stages.
1. Identification of relevant stratums and ensuring their actual representation in the population. Apart from gender as illustrated in example above, range of criteria that can be used to divide population into different strata include age, the level of education, status, nationality, religion and others. Specific patterns of categorization into different stratums depend on aims and objectives of the study.
In our case, BMW Group employees are employed across four business segments – automotive, motorcycles, financial services and other entities[1]. Accordingly, each segment can be adapted as stratum to draw sample group members.
2. Numbering each subject within each stratum with a unique identification number.
3. Selection of sufficient numbers of subjects from each stratum. It is critically important for samples from each stratum to be selected in a random manner so that the relevance of bias can be minimized. As it is illustrated in the table below, following the procedure described above results in the sample group of 16 respondents – BMW Group medium level managers that proportionately represent all four business segments of the company.
| Automotive | Motorcycles | Financial services | Other entities | |||||||||
| N | Manager | ü | N | Manager | ü | N | Manager | ü | N | Manager | ü | |
| 001 | Hudson | 001 | Conrad | ü | 001 | Guzman | 001 | Sparks | ||||
| 002 | Bass | ü | 002 | Braun | 002 | Craig | 002 | Atkinson | ü | |||
| 003 | Richmond | 003 | Gentry | 003 | Green | ü | 003 | Montes | ||||
| 004 | Tucker | 004 | Hartman | ü | 004 | Ballard | ü | 004 | Mcguire | |||
| 005 | Chavez | ü | 005 | Levine | 005 | Cox | 005 | Spencer | ü | |||
| 006 | Riddle | 006 | Griffin | ü | 006 | Dunlap | ü | 006 | Davies | |||
| 007 | Mckinney | 007 | Valentine | 007 | Patrick | 007 | Bradford | ü | ||||
| 008 | Terrell | ü | 008 | Mcdonald | 008 | Gardner | ü | 008 | Collins | |||
| 009 | Hayes | 009 | Brown | ü | 009 | Carpenter | 009 | Chen | ||||
| 010 | Escobar | ü | 010 | Kaufman | 010 | Vasquez | 010 | Hess | ü | |||
Advantages of Stratified Sampling
- Stratified random sampling is superior to simple random sampling because the process of stratifying reduces sampling error and ensures a greater level of representation.
- This sampling method captures key characteristics of population in the sample.
- Thanks to the choice of stratified random sampling adequate representation of all subgroups can be ensured.
- When there is homogeneity within strata and heterogeneity between strata, the estimates can be as precise (or even more precise) as with the use of simple random sampling.
Disadvantages of Stratified Sampling
- The application of stratified random sampling requires the knowledge of strata membership a priori. The requirement to be able to easily distinguish between strata in the sample frame may create difficulties in practical levels.
- Overlapping issues may occur in a way that some subjects may fall into different subgroups. This can result in misrepresentation of the population.
- Research process may take longer and prove to be more expensive due to the extra stage in the sampling procedure.
- The choice of stratified sampling method adds certain complexity to the analysis plan.
My e-book, The Ultimate Guide to Writing a Dissertation in Business Studies: a step by step approach contains a detailed, yet simple explanation of sampling methods. The e-book explains all stages of the research process starting from the selection of the research area to writing personal reflection. Important elements of dissertations such as research philosophy, research approach, research design, methods of data collection and data analysis are explained in this e-book in simple words.
John Dudovskiy